System MTTF and Failure Rates with No Maintenance
Whenever an expression for system reliability can be obtained, a corresponding value for system MTTF can also be obtained. This is done by using the relationship:

where the integral ranges from (mission time) = zero to infinity. For systems having a constant failure rate , the expression for system reliability, , is given by:

However, if λSis not constant, then MTTF does not equal 1/λS.
As an example of a non-constant failure rate system, consider a system comprising two sub-systems that are parallel in terms of reliability (Figure 4-3).

The reliability of this system is given by:
Provided that the sub-system failure rates are constant, the system MTTF is given by integrating equation 2.10 in Chapter 2, General Philosophy and Process of Reliability Prediction, from to infinity. We thus obtain:

This quantity depends only on failure rates and not on time. The system failure rate, λS, on the other hand is given by:
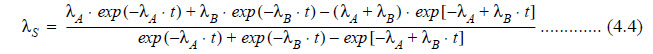
Or, if all of the failures are equal:

Note that both the above expressions for λS involve the variable (time).
Taking as an example λ=1000 fits (1 fit = 1 failure per 109 component hours), a plot λS of against time takes the form illustrated in Figure 4-4.
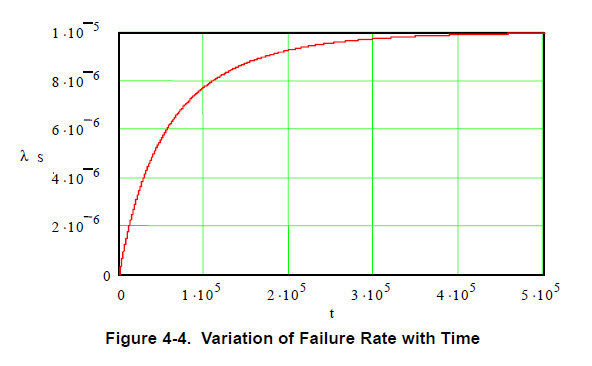
 (See
(See