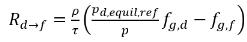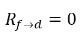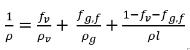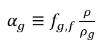Theory of Cavitation Models
In the vapor transport
equation 2.169, R
e and R
c are the mass transfer source terms connected to the growth and collapse of the vapor bubbles in cavitating flows. These terms account for the mass exchange between the vapor and liquid phases during a cavitation process. R
e and R
c are modeled on the
Rayleigh-Plesset equation describing the growth of a single vapor bubble in a liquid.
Liquid-Vapor Mass Transfer
To derive an expression of the net phase change rate in cavitation, consider an incompressible liquid-vapor two phase flow with zero-slip velocity where noncondensable gas is not considered. If you introduce R to represent the net rate of the mass transfer from the liquid to vapor, then you have the liquid and vapor volume fraction equations and the total mass continuity equation as follows:
• Liquid Phase
equation 2.179
• Vapor Phase
equation 2.180
• Mixture (Total Mass Continuity)
equation 2.181
In the liquid-vapor two-phase system, mixture density ρ is expressed in terms of the vapor volume fraction and phase densities:
equation 2.182
Since both the liquid and vapor density are assumed to be constant (incompressible), a relation between the velocity gradient and vapor volume fraction is derived from
equation 2.181 and
equation 2.182:
equation 2.183
Combining
equation 2.179 and
equation 2.183 gives the expression for net mass source term as:
equation 2.184
Substituting
equation 2.184 into
equation 2.180, the equation for the vapor volume fraction is rephrased in the general form:
equation 2.185
By applying the relation between the mass fraction and volume fraction of the vapor
equation 2.173, you have
equation 2.185 in terms of the vapor mass fraction:
equation 2.186
From
equation 2.185 and
equation 2.186, it is understood that under the condition of zero velocity slip between the liquid and vapor phase, cavitation is modeled as a single-phase flow with an additional vapor mass fraction transport equation, or as a
Eulerian multiphase mixture flow with liquid-vapor mass transfer. Without considering the effect of diffusion and phase velocity difference, the two approaches are identical mathematically.
Creo Flow Analysis uses the single-phase approach to model cavitating flows.
Bubble Dynamics Consideration
For most natural occurrences and engineering systems, there are an adequate number of nuclei such as bubbles, noncondensable gases, and so on in the liquid for cavitation inception. To model the cavitation process, consequently, the focus is primarily on correctly accounting for the bubble growth and collapse. Assuming that in a flowing liquid there is zero velocity slip between the liquid and vapor bubbles, the bubble dynamics equation is derived from the generalized Rayleigh-Plesset equation that describes the growth of a gas bubble in a liquid:
equation 2.187
where,
RB | bubble radius |
pB | pressure in the bubble (assumed to be the vapor pressure at the liquid temperature in the absence of other gases) |
p | pressure in the liquid surrounding the bubble |
σ | surface tension coefficient between the liquid and vapor |
This equation is derived from the mechanical balance (no thermal barriers to bubble growth).
If you neglect the second order time derivative (appropriate for low oscillation frequencies), the viscous damping term, and surface tension force, you have a reduced expression of
equation 2.187 that is valid for the asymptotic state:
equation 2.188
This reduced
Rayleigh-Plesset equation provides a physical approach to introduce the effects of bubble dynamics into cavitation models. The bubble radius can increase or decrease depending on the signs of (p
B–p): the bubble grows if p<p
B and collapses when p>p
B. Therefore,
equation 2.188 is rewritten as follows:
equation 2.189
If η is the vapor bubble number density in a liquid (the number of bubbles present per unit volume), and all the vapor bubbles are perfect spheres with the same radius RB, then the vapor phase volume fraction is as follows:
equation 2.190
It is assumed that the vapor bubbles cannot be created or destroyed in a liquid, but the bubbles can grow (evaporation) and collapse (condensation) during a cavitation process. In
equation 2.190, the vapor bubble number density (η) remains a constant, but the bubble radius (R
B) increases or decreases. Then the time derivative of vapor volume fraction is calculated as
equation 2.191
Substituting
equation 2.191 into
equation 2.186 gives the transport equation governing the vapor phase mass fraction:
equation 2.192
By applying
equation 2.189, the net rate of mass transfer per unit volume between the liquid and vapor has the following form:
equation 2.193
where
equation 2.193 indicates that in cavitation, the unit volume mass transfer rate (R) is the function of (proportional to) both vapor and liquid phase density and is also inversely proportional to the mixture density. Since
equation 2.192 is derived directly from the phase and mixture mass continuities, it is exact and should accurately represent the mass transfer between the liquid and vapor phase in cavitation. With the introduction of bubble dynamics,
equation 2.193 uses the similar approach to model the two opposite and physically different mass transfer processes: from liquid to vapor (bubble growth or evaporation), and from vapor to liquid (bubble collapse or condensation). For the vapor mass fraction transport
equation 2.192, the bubble growth is a source term, while the bubble collapse is treated as a sink term.
In practical cavitation models, the local far-field pressure p is usually taken to be the same as the cell center pressure. Bubble pressure pB is equal to saturation vapor pressure (psat, a material property) in the absence of dissolved gases, mass transport, and viscous damping, pB=psat
If you compare
equation 2.192 and
equation 2.193 with the general vapor mass fraction
equation 2.169, the source terms R
e and R
c are as follows:
• If the local flow pressure is below the saturation vapor pressure, p<psat, only evaporation occurs so that:
equation 2.194
• If the local flow pressure is above the saturation vapor pressure p>psat, there is only condensation:
equation 2.195
Equation 2.194 and
equation 2.195 form the basis of almost all the available mechanistic two-phase cavitation models.
Creo Flow Analysis has used the
Singhal et al modeling approach.
Gas Absorption/Dissolution and Release
Noncondensable gases are often present in a working fluid and can have significant impact on cavitation. Sometimes a noncondensable gas freely transports with the flow, and also dissolves into or releases from a liquid, naturally aiming towards a dynamic equilibrium of the mass concentrations between the liquid and gas phases. The gas absorption or dissolution and release in a liquid is also a liquid-gas mass transfer phenomenon, which is driven by the mass concentration differences and gradients. To model cavitating flows it is necessary to also account for the effect of the noncondensable gas and the possible liquid-gas mass transfer in the mixture flow.
Assuming that in a liquid-gas two phase flow, a noncondensable gas such as air, or oxygen, exists in both liquid phase (dissolved gas) and gas phase (free gas), the gas mass fraction transport equations in each phase follows:
• Free Gas (gas phase)
equation 2.196
• Dissolved Gas (liquid phase)
equation 2.197
where,
fg,f | mass fractions of the free gas |
fg,d | mass fractions of the free gas and dissolved gas, |
Sg,f, Sg,d | external or user-defined sources. |
Dg,f | diffusivity of the free gas and dissolved gas |
Dg,d | diffusivity of the dissolved gas |
If mass fraction of a noncondensable gas is predescribed as fg, then you have:
fg=fg,f
or its transport in space and time are obtained by solving the following equation:
equation 2.199
In
equation 2.196 and
equation 2.197, source R
d→f indicates release rate of the dissolved gas and R
f→d indicates absorption or dissolution rate of the free gas.
When the two phases are in contact, there is a tendency for the free gas f and dissolved gas d transporting from one phase to the other to achieve a dynamic equilibrium between the two phases. The equilibrium models assume that the volumetric rates of the mass transfers depend on the mass-concentration gradients or differences:
equation 2.200
equation 2.201
where,
AI | liquid-gas interfacial area |
kf,d(=kd,f) | bulk volumetric mass transfer coefficient |
ρg,d(=ρfg,d) | local mass concentrations of dissolved gas |
ρg,f(=ρfg,f) | local mass concentrations of free gas |
| equilibrium mass concentrations of the dissolved gas on its hosting phases |
| equilibrium mass concentrations of the free gas on its hosting phases |
Note that
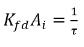
has the unit of inversed time, 1/s, an indicator of the mass transfer efficiency. Therefore,
equation 2.200 and
equation 2.201 also have the following forms:
equation 2.202
equation 2.203
Typically
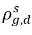
and

are not the same (discontinuity). There is a well-defined equilibrium curve between the two concentrations, which depends on temperature, pressure, and mixture compositions. The curve is usually monotonic and nonlinear, and is often expressed as a quasi-linear relation with coefficient

equation 2.204
where K
f,d is usually decided using physical laws or empirical correlations. One common approach is to follow
Henry’s law that provides a generalized equilibrium relationship. This states that for a liquid mixture in contact with the gas phase, the partial pressure of the free gas ρ
g,f is equal to the product of the equilibrium mole fraction of the dissolved gas in the liquid phase,
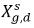
and the Henry constant, H
x:
equation 2.205
If the free gas phase follows the Ideal Gas Law, then Dalton’s law of partial pressure gives the following equation:
equation 2.206
equation 2.207
Therefore, Henry’s constant has a unit of pressure and can be considered as a reference pressure. Assuming, for an ideal liquid mixture in contact with a gas, Henry’s constant is the saturation vapor pressure p
sat, then
equation 2.207 is also given as:
equation 2.208
where p
d,equil,ref is the reference pressure for the dissolved equilibrium mass fraction. Then
equation 2.204 is rewritten as:
equation 2.209
In
equation 2.202 and
equation 2.203,
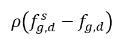
and

are the mass concentration differences/gradients, the driving forces of the absorption/dissolution and release of the noncondensable gases. It indicates that the noncondensable gas transport across two phases requires a departure of mass concentration from its equilibrium state. The direction of the mass transfer moves the system towards equilibrium depending on local and equilibrium mass concentrations on both phases. From the equilibrium model, you have the following:
◦ Gas Absorption/Dissolution into a Liquid—Mass transfer takes place from the gas phase (free gas) to the liquid phase (dissolved gas). The equilibrium model assumes that the free gas at the gas phase is at the equilibrium state:
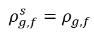
. Using
equation 2.204 and
equation 2.209, gives the following:
equation 2.210
equation 2.211
equation 2.212
◦ Gas Release from a Liquid—Mass transfer takes place from the liquid phase (dissolved gas) to the gas phase (free gas). In this process, the equilibrium model assumes that the dissolved gas in the liquid phase is always in the equilibrium state:

. From
equation 2.202,
equation 2.203,
equation 2.204, and
equation 2.209, the rates of mass transfers are as follows:
equation 2.213
equation 2.214
Note that when a part of the noncondensable gas is dissolved into the liquid, the freely expandable gas is only the portion remaining in the gas phase, fg,f. Hence the mixture density is calculated as:
equation 2.215
and the volume fraction of the free gas is as follows:
equation 2.216
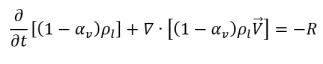
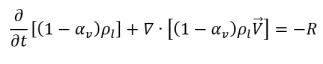
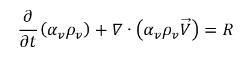
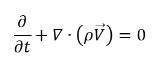

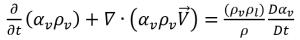
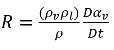
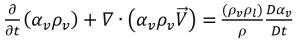

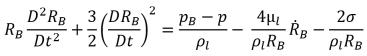
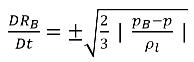
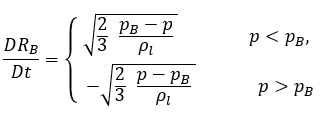
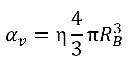
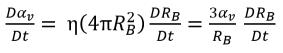
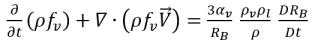
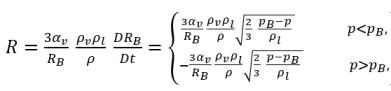
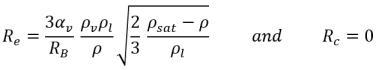
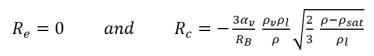
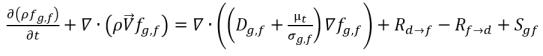
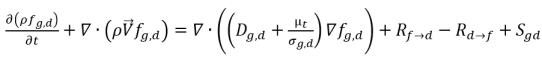
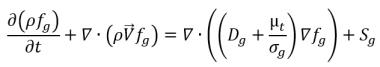
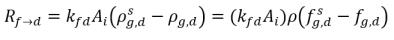
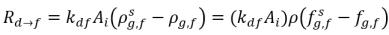
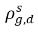
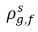
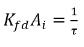 has the unit of inversed time, 1/s, an indicator of the mass transfer efficiency. Therefore, equation 2.200 and equation 2.201 also have the following forms:
has the unit of inversed time, 1/s, an indicator of the mass transfer efficiency. Therefore, equation 2.200 and equation 2.201 also have the following forms: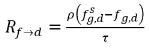
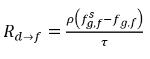
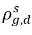 and
and  are not the same (discontinuity). There is a well-defined equilibrium curve between the two concentrations, which depends on temperature, pressure, and mixture compositions. The curve is usually monotonic and nonlinear, and is often expressed as a quasi-linear relation with coefficient
are not the same (discontinuity). There is a well-defined equilibrium curve between the two concentrations, which depends on temperature, pressure, and mixture compositions. The curve is usually monotonic and nonlinear, and is often expressed as a quasi-linear relation with coefficient 
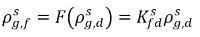
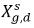 and the Henry constant, Hx:
and the Henry constant, Hx: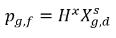
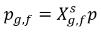
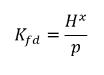
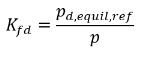

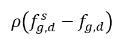 and
and  are the mass concentration differences/gradients, the driving forces of the absorption/dissolution and release of the noncondensable gases. It indicates that the noncondensable gas transport across two phases requires a departure of mass concentration from its equilibrium state. The direction of the mass transfer moves the system towards equilibrium depending on local and equilibrium mass concentrations on both phases. From the equilibrium model, you have the following:
are the mass concentration differences/gradients, the driving forces of the absorption/dissolution and release of the noncondensable gases. It indicates that the noncondensable gas transport across two phases requires a departure of mass concentration from its equilibrium state. The direction of the mass transfer moves the system towards equilibrium depending on local and equilibrium mass concentrations on both phases. From the equilibrium model, you have the following: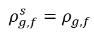 . Using equation 2.204 and equation 2.209, gives the following:
. Using equation 2.204 and equation 2.209, gives the following: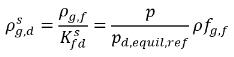

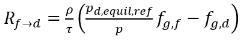
 . From equation 2.202, equation 2.203, equation 2.204, and equation 2.209, the rates of mass transfers are as follows:
. From equation 2.202, equation 2.203, equation 2.204, and equation 2.209, the rates of mass transfers are as follows: