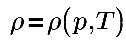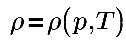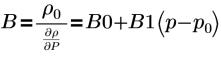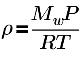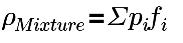Definitions
• Density—A material property that defines the state of a fluid flow as incompressible or compressible. The constitutive equation of state for density has the general form:
where,
In addition to the user-defined density law using expression editor, several special constitutive equations of state are given to determine the flow density in order to close the above governing equations.
• Constant Density—In a fluid flow, the assumption of constant density simplified the flow as incompressible. For most liquids, the density is considered as a constant unless it is under significantly large variations of pressure, temperature, or both pressure and temperature.
• Compressible Liquid—In water hammers and fuel injectors, the compressibility effects of a liquid are essential to determine the flow characteristics and therefore the performances of the flow components, equipment, or both flow components and equipment. The following compressible liquid model is available in Creo Flow Analysis:
• Ideal Gas Law—For compressible flows, the ideal gas law relates the gas density to the absolute flow pressure and temperature in the following form:
where,
P | Absolute pressure expressed using N/m2(gauge pressure + operating/reference pressure) |
T | static temperature (Kelvin) |
Mw | molecular weight |
R | universal gas constant |
• Isentropic Gas Law—In Flow Analysis, it is the absolute temperature Kelvin (K). In terms of thermodynamic properties, temperature is the driving potential for energy transfer as heat.
where,
ρt | reference density |
Pt | reference pressure |
Tt | reference temperature |
γ=CP/CV | Adiabatic index, ratio of Specific Heat at constant pressure to the Specific Heat at constant volume |
M | Mach number |
• Mass Fraction Average—A method for computing the average density for a multicomponent mixture in a selected volume.
where,
ρi | density |
fi | concentration of the individual components respectively |
• Volume Fraction—Volume of a component divided by the total volume within a selected volume or at a point.