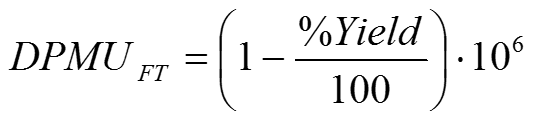The variation plot shows the worst-case range and statistical distribution for the measurement. You can use the shortcut menu for the variation plot to turn off the display of the statistical or worst-case plot.

The measurement specification limits and the target value are user-specified values. These define the design requirements for the measurement. The statistical variation is represented by a statistical distribution. Creo Tolerance Analysis powered by CETOL Technology supports three distribution types: Normal, Uniform, and Lambda. The distribution moments depend on the distribution type, which is determined based on best-fit criteria. For most real-life problems, the results will be represented by either a normal or lambda distribution.

The table below shows the formulas used to calculate quality metrics.

The value for Sigma is calculated by first calculating first time dpo (defects per unit opportunity), and then finding a one-sided Z-score that has the same first time dpo. A one-sided Z-score is the value of a unilateral limit for a normal distribution with a mean equal to zero and standard deviation equal to one.